Mathematical Models (Equations)
 |
| Area Model [5th Grade] Add it up! I hope teachers toss out this junk and focus on standard algorithms. |
What has happened to simple arithmetic? The uncomplicated answer is that inferior methods from reform math have been in vogue for decades. For example, in reform math via State Standards and Common Core, students are often asked to make drawings, such as the area model (5.3 x 2.4), to calculate or justify their math, which I think is confusing, pointless, and useless for novices. Indeed, popular reform math methods make simple arithmetic unduly and ridiculously complicated.
The modern "visual" approach, such as the area model (left), in my opinion, wastes valuable classroom time on pointless material that leads nowhere. Put simply, no one calculates this way! Why should kids labor over nonessentials? ( In contrast to American math instruction, kids in top-performing nations drill and memorize important math facts and practice for mastery efficient procedures for abstract operations starting in the 1st-grade. Indeed, 1st-graders in Singapore carry and borrow (regroup) in standard addition and subtraction calculations, do multiplication as repeated addition and write equations in three operations--addition, subtraction, and multiplication--from word problems. Our 1st-graders do not come close. )
The "visual" approach seems confusing and needlessly complex. It inhibits the learning of standard arithmetic by restricting the time spent on learning the standard algorithms. Standard algorithms are often minimized and portrayed as merely one of many ways to calculate--even discouraged in many classrooms. The efficient use of standard algorithms requires the memorization of single-digit math facts in long-term memory. Reformers claim that "sketching visuals" is needed to show understanding or as justification for answers, which is reform math hype. Regrettably, the importance of numerical relationships and their symbolic representations in mathematical language, which are important for understanding standard arithmetic, have been undervalued, trivialized, and delayed. Children are novices; they need to memorize single-digit math facts, practice standard algorithms for mastery, use mathematical language, and mathematize word problems.
The symbols of math, detached from physical content, and the abstract operations of arithmetic are the very essence of algebra. Abstract rules, such as a + b = b + a, or (a + b) + c = a + (b + c), or a(b + c) = ab + ac, and others, govern all of arithmetic, including the standard algorithms. "The strength of arithmetic lies in its absolute generality. Its rules admit of no exceptions: they apply to all numbers," writes Tobias Dantzig (Number, 1930).
Furthermore, an important part of understanding arithmetic-that-leads-to-algebra is the ability to do arithmetic quickly, efficiently, and effortless. Students should avoid doing calculations via complicated visuals or drawings (extra baggage), such as the area models, array models, charting models, bar models, or other drawings. In contrast, to do simple arithmetic, the student should use fast, efficient procedures that are the standard algorithms. The drawings often become the focus, and they distract from the straightforward mastery of basic arithmetic needed to advance to algebra and beyond. Doing math well is doing it as simply and as efficiently as possible, which is contrary to reform math methods. Reform math apostles argue that students understand math only when they can make a "drawing" or write an "explanation" as a justification for an answer. They are wrong!
In my opinion, instructional time is better spent on standard arithmetic, writing equations and finding solutions to solve problems (aka mathematizing). It is important for students to express numerical relationships in abstract, mathematical language, such as y = 3x - 1.
The understanding of mathematics is rooted in the meaning of symbolic mathematical language, not in drawing visuals, etc. It is rooted in the abstract, not in the concrete.
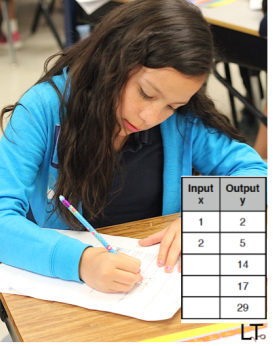 |
| The 4th-grade student figured out the rule and wrote an equation. |
Basic algebra is accessible to very young children when it is fused to the fundamentals of standard arithmetic. My "early algebra" program (Teach Kids Algebra) is an attempt to do this in actual classrooms. I use x-y tables as a stepping stone for figuring out function rules that lead to writing equations in two variables. Students can find function rules to complete x-y tables, write linear equations, work backward with inverse concepts (undo) to find x when given y, and graph tables on a coordinate plane. The key part is writing an equation. I have used function rules and building tables as methods for writing equations as early as 1st-grade in my Teach Kids Algebra program. By 4th-grade, the equations are more difficult (See table: left). The inverse concept is an important and useful mathematical concept, not only for table building but also for solving equations. Unfortunately, inverses are seldom taught in early elementary school. It seems that educators think that inverse ideas to solve equations are too advanced. I disagree. Even 1st-grade students can understand simple inverse ideas: 6 + 4 - 4 = 6 or n - 5 + 5 = n. It is simple to demonstrate and easy to learn by reasoning.
"Mathematical expressions and sentences [equations] can be applied to real life situations to describe numerical relationships. The same mathematical expression or sentence [aka equation] may represent the numerical facts in more than one situation." (Dolciani & Wooton, Modern Algebra, 1970 ) The numerical relationships are mathematical models.
[Special Note. Reform math people think the best way to make sense of math is through creating visuals, such as charts, graphs, and diagrams, not symbolic representations. I think this is superficial because students should focus on the symbolic representation, not drawings. The reformers claim a better way to learn algebra is through tables and graphs made on a graphing calculator. If that were true, then our students would be the best algebra students in the world. Unfortunately, the graphing calculator is required for most algebra classes and the SAT. The overuse of graphing calculators to solve problems in algebra textbooks is often at the expense of symbolic representations and manipulation.
What is important, I think, is that young students use mathematical language to write expressions and equations and use algebra concepts to solve equations. Too often, our math programs do not stress abstract, symbolic representation enough. Moreover, we should not insist that students make drawings or write an explanation to justify answers. Students need to mathematize word problems via the language of math (aka equations). Then, they need to apply algebra concepts (inverses) to solve the equations. In short, students need to write and solve equations. For example, my 1st-grade students figured out function rules, wrote equations, built tables, and plotted the graphs in Q-1.]
What is important, I think, is that young students use mathematical language to write expressions and equations and use algebra concepts to solve equations. Too often, our math programs do not stress abstract, symbolic representation enough. Moreover, we should not insist that students make drawings or write an explanation to justify answers. Students need to mathematize word problems via the language of math (aka equations). Then, they need to apply algebra concepts (inverses) to solve the equations. In short, students need to write and solve equations. For example, my 1st-grade students figured out function rules, wrote equations, built tables, and plotted the graphs in Q-1.]
Mathematical models are written in mathematical language: symbols for the known numbers, the unknown (variables), operations, calculations, solutions, etc. Furthermore, mathematical models imply a level of understanding, knowledge, and skills, which are substantially better than making visuals or writing an explanation as an afterthought. Students must be able to convert (mathematize) a word problem into mathematical language correctly. In short, the student must be able to abstract what is known (the numbers), the unknown, the operations needed, and then synthesize them into a coherent whole, that is, an equation that corresponds to or models directly the word problem.
 |
| Writing equations in the 1st grade to describe word problem situations is a key skill. |
Writing and solving equations that lead directly to a correct solution requires adequate (prerequisite) mathematical knowledge and thinking. The steps are enough to imply understanding. Indeed, writing an equation and solving it in a sequence of logical steps to find the unknown is the root of understanding in mathematics.
To mathematize a word problem, students need to separate the known numbers, the operation(s) needed, and the unknown from the words and then put the symbols together in an equation that corresponds to the problem and, when solved or calculated, leads to a correct solution.
Teaching children to write and think in mathematical language is not an easy task. Traditionally, mathematizing has been a troublesome area in our math programs. Still, kids must learn to write and think systematically in mathematical language, beginning in 1st-grade, which is when Singapore children start to write equations in one variable.
Note. In the equation n + 12 = 45, to undo add 12, subtract 12 from both sides of the equation to isolate n and maintain the balance (equality). Algebra focuses on equations. It is vital that young children turn sentences or word problems into math language (aka an equation) and practice for mastery the algebra techniques for solving equations, which means to isolate the variable. To isolate the variable is to get the variable n by itself on one side of the equation, such as n = 33. To do it (method), we undo operations. "For the two sides of the equation to stay equal, whatever we do to one side has to be done to the other."
-------------------------------
Sample 3rd-Grade Problem
Sally has some pencils. Ben gives her 79 more pencils. Now Sally has 167 pencils. How many pencils did Sally have at the beginning? (Think this way: The known numbers are 79 and 167, the operation is +, and the unknown is n. With these abstract symbols (aka math language) think up an equation that closely models the word problem and leads to a correct solution when solved. See below.)
Mathematical Models
Applying Mathematical Language & Thinking
n + 79 = 167
n + 79 = 167 (calculating, solving)
-79 -79
n + 0 = 167 - 79
n = 88
88 + 79 = 167 (checking)
167 = 167 (true)
The model in mathematical language becomes 88 + 79 = 167.
The steps shown above imply adequate understanding.
Drawings or explanations are not needed or helpful.
The steps shown above imply adequate understanding.
Drawings or explanations are not needed or helpful.
Nonmathematical Language: Sally started with 88 pencils.
-----------------------------------
Note Well. First-grade and second-grade students should start with true/false concepts, guess and check, rule for substituting, and memorized math facts to solve simple equations, such as x + x - 3 = 7, but experienced 2nd-grade and most 3rd-grade students should leave guess and check behind and advance to a fundamental algebra idea that equations can be solved by "undo" operations (inverses) to isolate the variable. Thus, for the equation n + 79 = 167, to undo add 79, subtract 79. Insist that students show the steps and standard calculations needed to communicate and express their understanding. Addition and subtraction are opposites or inverses of each other and undo each other. Thus, n + 79 - 79 is n + 0 or n. Also, the operation of subtracting 79 must be applied to both sides of the equation to keep the equation balanced (equal). Multiplication and division are inverses, too, and undo each other. When working with equations, students should always Think Like A Balance.
Note. The model 88 + 79 = 167 applies to many different mathematical situations, including the pencil problem above. It is the power of abstraction in mathematics. A simple math fact, such as 2+3=5, is a mathematical model but, because it is commonplace, we do not think of it as a model. Despite its simplicity, the 2+3=5 addition fact is a very powerful model and applies to many different concrete situations found in the real world, which is the point the late Morris Kline makes in his book Mathematics for the Nonmathematician, 1967.
[Aside. Morris Kline, a mathematician, writes, "When a child learns that 5 + 5 = 10 [or 36 ÷ 9 = 4, etc.], he acquires in one swoop a fact which applies to hundreds of situations. Part of the secret of the power of mathematics is that it deals with abstractions. Whole numbers and fractions and the various operations with whole numbers and fractions are abstractions."]
Put simply, arithmetic is abstract and should be taught through conceptual symbols.
Its understanding is rooted in abstract, symbolic language. The problem with arithmetic today is that we have gotten away from its symbolic structure and substituted pseudo-mathematical models and thought such as the area model or the array model.
Unfortunately, we have underestimated the key importance of standard algorithms (fast, efficient procedures), which are part of the wonderful, abstract tapestry of mathematics, and the memorization of single-digit math facts for auto recall in problem-solving, which is a basic tenet of cognitive science (the relationship between working memory and long-term memory). Practice and memorization are part of learning the structure and method of arithmetic and algebra.
Put simply, for decades we have been teaching math poorly. The reason is that progressive reform math has been taught while tried-and-true standard arithmetic, memorization, and practice have been deemphasized. We need to restore traditional arithmetic, both structure and methods, that prepares students for algebra in middle school.
----------------------------
[Extra. Demonstrate that the expressions 56 • 1/8 and 7 are equivalent by stating the reason that justifies each step. Showing the steps implies adequate understanding. The steps, themselves, are enough; however, students should also know the reasons that govern each step, so they do correct mathematics.
An equation consists of two expressions set equal to each other. The rules of arithmetic and algebra consist of assumptions, principles, definitions, axioms, properties, and conventions. Some equations contain variables such as x + 3x = 200. The coefficients of x and 3x are 1 and 3 respectively. We do not write 1x just x. Also, 3x is a product and means 3 • x or x + x + x. Students must know the rules and use them. Expressions and equations show numerical relationships.]
-------------------------------
Dr. Katharine Beals (Out in Left Field), summarizes reform math: "Along with group work, group discovery, multiple solutions, and, of course, explaining answers to easy problems, there's doing math visually." I think Beals is right. The problem with all this is that kids do not learn much content. Early on, elementary students are not required to work with abstract, symbolic representations, which are so vital to understanding arithmetic and algebra.
Relying too much on visual representations often downplays the importance of mathematical language that is the writing of equations from word problems (mathematizing), the use of algebra concepts to solve the equations, and the learning of conceptual symbols to do arithmetic. The problem starts in the lower elementary school where students use counting strategies (via visuals, pictures, manipulatives, etc.) to do simple arithmetic. I am disturbed that students are often required to make a drawing for a world problem or write an explanation for easy arithmetic. The meaning of mathematics is rooted in mathematical language, not in making visuals, etc. It is rooted in the abstract, not in the concrete.
To Be Revised
Last update: 11-24-15, 11-28-15, 11-29-15, 11-30-15
Credits
Model: Jayne
Some ideas of mathematizing from Numbers by Alfred S. Posamentier & Bernd Thaller
The idea of showing that 5 •1/8 and 7 are equivalent expressions is from Modern Algebra by Dolciani & Wooton
Area Model from Kaplan: A Parent's Guide to the Common Core, Grade 5
Some ideas of mathematizing from Numbers by Alfred S. Posamentier & Bernd Thaller
The idea of showing that 5 •1/8 and 7 are equivalent expressions is from Modern Algebra by Dolciani & Wooton
Area Model from Kaplan: A Parent's Guide to the Common Core, Grade 5
